ζ Pup: XMM RGS line-profile analysis
Fe XVII at 16.780 A
For this line we use both the RGS1 and RGS2 data, and as with the other lines, we do not allow shift to be a free parameter. There is mild blending with the blue wing of the 17.05/17.10 complex. We eliminate a large portion of the red wing of the 16.780 line from the fit, and also include a model component for the line near 17 A (although with the red wing eliminated from the fit, the inclusion of the 17A model does not make a difference at all).
First we repeat the non-porous fit shown on the main page.
Non-porous: RGS1+2
RGS1
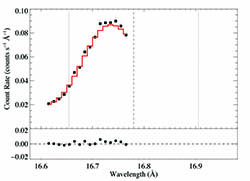
RGS2
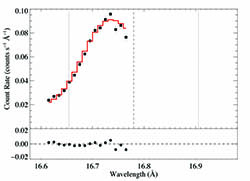
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.19e-3 +/- (:)
q = 0
hinf = 0
taustar = 3.38 +/- (2.93:3.69)
Ro = 1.54 +/- (1.15:1.87)
shift = 0
norm = 3.01e-4 +/- (2.95e-4:3.08e-4)
chisq = 30.34 for N = 32
|
We show the two spectra separately, but they were fit simultaneously. That's the same model in both plots.
Compare these results to the same sort of model fit to the Chandra spectrum. The results are consistent.
Next, we'll fit models with anisotropic porosity assuming flattened, radially oriented clumps. We use the "stretch" porosity length distribution, h(r), parameterized by hinf. We will fit first with hinf a free parameter, and then at fixed values of hinf = 0.5, 1, 2, and 5 R*.
aniso-porous: RGS1+2, hinf free
RGS1
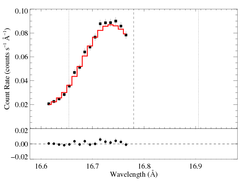
RGS2
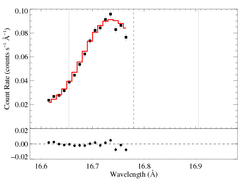
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.19e-3 +/- (:)
q = 0
hinf = 0.00 +/- (0.00:0.02)
taustar = 3.38 +/- (2.93:3.69)
Ro = 1.54 +/- (1.30:1.87)
shift = 0
norm = 3.01e-4 +/- (2.95e-4:3.08e-4)
chisq = 30.34 for N = 32
|
A best-fit value of hinf = 0 is found, recovering the non-porous fit. However, because the porosity length is allowed to be a free parameter in this fit, the confidence limits could be different (and larger), so we show the details, above, though they turn out to be very similar to the confidence limits for the non-porous model fit (probably because even tiny non-zero porosity lengths are ruled out).
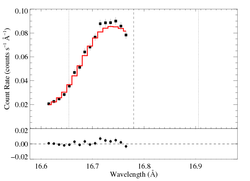
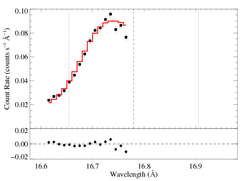
aniso-porous: RGS1+2, hinf = 0.5
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.03e-3 +/- (1.79e-3:2.27e-3)
q = 0
hinf = 0.5
taustar = 4.01 +/- (3.18:4.84)
Ro = 1.99 +/- (1.74:2.25)
shift = 0
norm = 3.20e-4 +/- (3.13e-4:3.27e-4)
chisq = 42.17 for N = 32
|
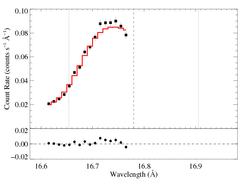
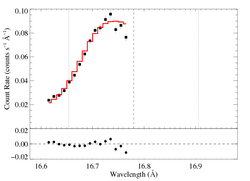
aniso-porous: RGS1+2, hinf = 1
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 1.98e-3 +/- (1.79e-3:2.27e-3)
q = 0
hinf = 1
taustar = 4.17 +/- (3.13:5.43)
Ro = 2.24 +/- (1.99:2.48)
shift = 0
norm = 3.29e-4 +/- (3.22e-4:3.37e-4)
chisq = 48.01 for N = 32
|
aniso-porous: RGS1+2, hinf = 2
RGS1
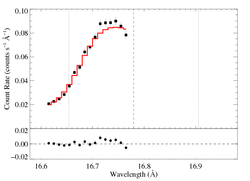
RGS2
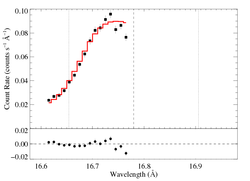
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 1.93e-3 +/- (1.68e-3:2.17e-3)
q = 0
hinf = 2
taustar = 4.47 +/- (2.84:6.34)
Ro = 2.49 +/- (2.25:2.72)
shift = 0
norm = 3.41e-4 +/- (3.33e-4:3.50e-4)
chisq = 54.46 for N = 32
|
aniso-porous: RGS1+2, hinf = 5
RGS1
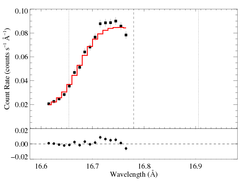
RGS2
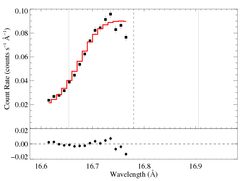
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 1.87e-3 +/- (:)
q = 0
hinf = 5
taustar = 4.28 +/- (:)
Ro = 2.89 +/- (:)
shift = 0
norm = 3.59e-4 +/- (:)
chisq = 62.29 for N = 32
|
Summarizing
The effect of porosity and the tradeoff between porosity length and optical depth can be summarized by looking at the joint parameter confidence limits. We show the 68%, 95%, and 99% limits below. The best-fit model is the filled circle.
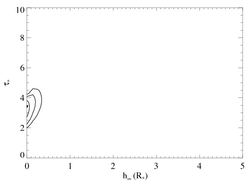
Now for models with isotropic porosity.
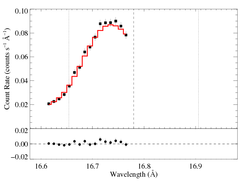
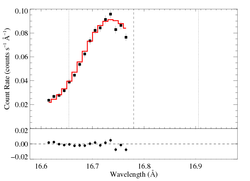
iso-porous: RGS1+2, hinf free
As with the anisotropic case, allowing the terminal porosity length to be a free parameter leads to a best-fit hinf = 0, and we recover the non-porous global best-fit model. We show it here, including the parameter confidence limits which are generally larger than found for the non-porous model.
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.19e-3 +/- (:)
q = 0
hinf = 0.00 +/- (0.00:0.48)
taustar = 3.34 +/- (2.98:4.17)
Ro = 1.57 +/- (1.18:1.86)
shift = 0
norm = 3.01e-4 +/- (2.95e-4:3.08e-4)
chisq = 30.32 for N = 32
|
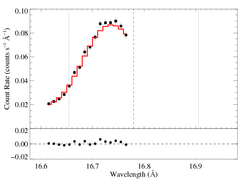
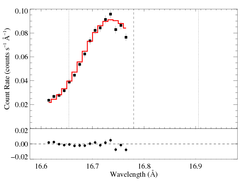
iso-porous: RGS1+2, hinf = 0.5
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.16e-3 +/- (1.93e-3:2.39e-3)
q = 0
hinf = 0.5
taustar = 3.94 +/- (3.31:4.50)
Ro = 1.65 +/- (1.42:1.91)
shift = 0
norm = 3.01e-4 +/- (2.95e-4:3.08e-4)
chisq = 31.14 for N = 32
|
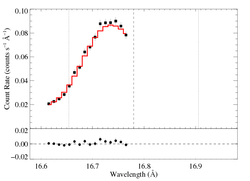
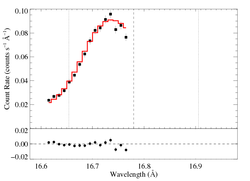
iso-porous: RGS1+2, hinf = 1
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.13e-3 +/- (1.90e-3:2.36e-3)
q = 0
hinf = 1
taustar = 4.50 +/- (3.67:5.39)
Ro = 1.73 +/- (1.52:1.96)
shift = 0
norm = 3.03e-4 +/- (2.96e-4:3.09e-4)
chisq = 32.19 for N = 32
|
Note: with hinf = 1, taustar has increased by 33 percent.
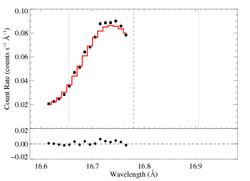
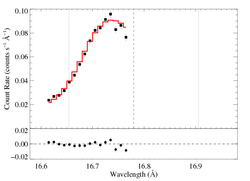
iso-porous: RGS1+2, hinf = 2
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 2.09e-3 +/- (1.85e-3:2.32e-3)
q = 0
hinf = 2
taustar = 5.61 +/- (4.44:7.14)
Ro = 1.86 +/- (1.68:2.06)
shift = 0
norm = 3.06e-4 +/- (2.99e-4:3.13e-4)
chisq = 34.44 for N = 32
|
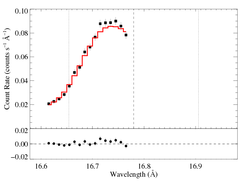
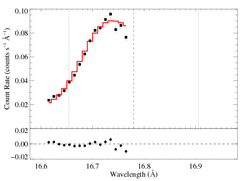
iso-porous: RGS1+2, hinf = 5
RGS1
RGS2
|
[16.60:16.77]
vinf = 2250
β = 1
powerlaw continuum, n = 2
norm = 1.99e-3 +/- (1.75e-3:2.23e-3)
q = 0
hinf = 5
taustar = 8.61 +/- (5.85:12.91)
Ro = 2.13 +/- (1.95:2.32)
shift = 0
norm = 3.19e-4 +/- (3.11e-4:3.26e-4)
chisq = 40.37 for N = 32
|
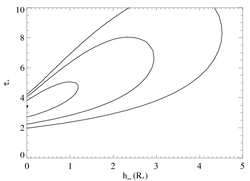
Summarizing
We show the 68%, 95%, and 99% limits below. The best-fit model is the filled circle.
And here is an extensive log of all these xspec fits.
Back to the top
last modified: 14 May 2012























