ζ Pup: XMM RGS line-profile analysis

Ne X Lyman-α at 12.134 A

We fit only the RGS2 data (there is very little RGS1 data, due to a camera failure -- the effects of including that additional data are shown in our archive of previous fits).

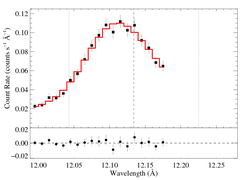
First we repeat the non-porous fit shown on the main page.

Non-porous: RGS2

[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.44e-3   +/- (1.14e-3:1.74e-3) q = 0 hinf = 0 taustar = 1.81   +/- (1.59:2.06) Ro = 1.61   +/- (1.43:1.76) shift = 0 norm = 3.10e-4   +/- (3.02e-4:3.18e-4) chisq = 24.04 for N = 19

This fit is formally pretty good (chi-sq = 24 for 15 d.o.f).

Compare these results to the analogous model fit to the same line in the Chandra spectrum. There is quite good agreement (taustar values disagree by only about 10%, well within the formal confidence limit range). The same is true for R_o, while the normalizations disagree by about 2-sigma.

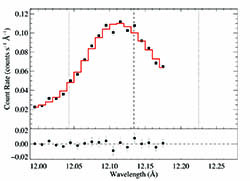
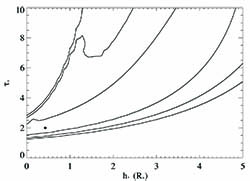
Next, we'll fit models with anisotropic porosity assuming flattened, radially oriented clumps. We use the "stretch" porosity length distribution, h(r), parameterized by h_inf. We will fit first with h_inf a free parameter, and then at fixed values of h_inf = 0.5, 1, 2, and 5 R_*.

aniso-porous: RGS2, h_inf free

[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.45e-3   +/- (:) q = 0 hinf = 0.00   +/- (0.00:0.28) taustar = 1.78   +/- (1.59:3.37) Ro = 1.62   +/- (1.01:1.77) shift = 0 norm = 3.10e-4   +/- (3.02e-4:3.19e-4) chisq = 24.06 for N = 19

Note that despite the fact that the terminal porosity length's best-fit value is found to be zero (1e-7 or so, actually), the best-fit model parameters are not identical to the non-porous fit. They are very close, of course, but the small differences are probably due to the numerical integration that's required in the aniso case. Note that the 68% upper confidence limit on h_inf is 0.28, and that enables larger values of taustar, and smaller values of R_o, apparently.

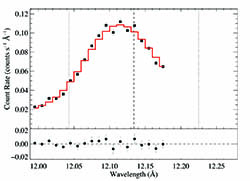
Now, a sequence of fits with fixed h_inf values of 0.5, 1, 2, and 5.

aniso-porous: RGS2, h_inf = 0.5

[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.15e-3   +/- (:) q = 0 hinf = 0.5 (frozen) taustar = 3.92   +/- (3.25:4.75) Ro = 1.35   +/- (1.16:1.56) shift = 0 norm = 3.18e-4   +/- (3.10e-4:3.26e-4) chisq = 27.27 for N = 19

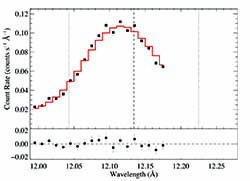
aniso-porous: RGS2, h_inf = 1

[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 9.70e-4   +/- (:) q = 0 hinf = 1 (frozen) taustar = 5.99   +/- (:) Ro = 1.42   +/- (:) shift = 0 norm = 3.23e-4   +/- (:) chisq = 34.22 for N = 19

aniso-porous: RGS2, h_inf = 2

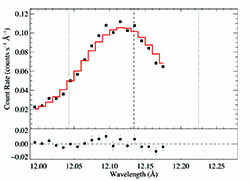
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 7.14e-4   +/- (:) q = 0 hinf = 2 (frozen) taustar = 12.61   +/- (:) Ro = 1.46   +/- (:) shift = 0 norm = 3.31e-4   +/- (:) chisq = 47.21 for N = 19

aniso-porous: RGS2, h_inf = 5

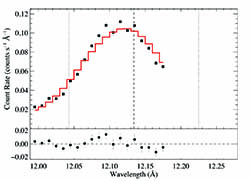
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 2.08e-4   +/- (:) q = 0 hinf = 5 (frozen) taustar = 64.82   +/- (:) Ro = 1.35   +/- (:) shift = 0 norm = 3.44e-4   +/- (:) chisq = 66.88 for N = 19

Summarizing

The effect of porosity and the tradeoff between porosity length and optical depth can be summarized by looking at the joint parameter confidence limits. We show the 68%, 95%, and 99% limits below. The best-fit model is the filled circle.

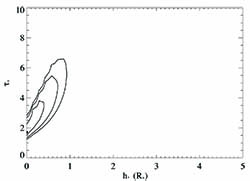
Now, we'll look at models with isotropic porosity.

iso-porous: RGS2, h_inf free

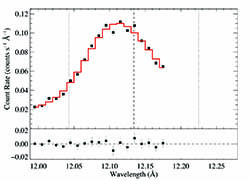
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.43e-3   +/- (:) q = 0 hinf = 0.33   +/- (0.00:3.83) taustar = 1.97   +/- (1.62:8.03) Ro = 1.61   +/- (1.41:1.77) shift = 0 norm = 3.10e-4   +/- (3.02e-4:3.18e-4) chisq = 24.04 for N = 19

Note poorly constrained h_inf and taustar.

Now, a sequence of fits with fixed h_inf values of 0.5, 1, 2, and 5.

iso-porous: RGS2, h_inf = 0.5

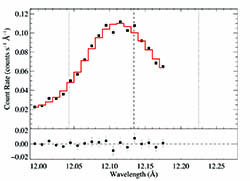
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.43e-3   +/- (:) q = 0 hinf = 0.5 (frozen) taustar = 2.07   +/- (1.80:2.41) Ro = 1.61   +/- (1.45:1.76) shift = 0 norm = 3.10e-4   +/- (3.02e-4:3.18e-4) chisq = 24.04 for N = 19

iso-porous: RGS2, h_inf = 1

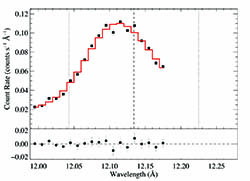
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.42e-3   +/- (:) q = 0 hinf = 1 (frozen) taustar = 2.41   +/- (2.07:2.86) Ro = 1.61   +/- (1.46:1.76) shift = 0 norm = 3.10e-4   +/- (3.02e-4:3.19e-4) chisq = 24.06 for N = 19

Note: with h_inf = 1, taustar has increased by 33 percent.

iso-porous: RGS2, h_inf = 2

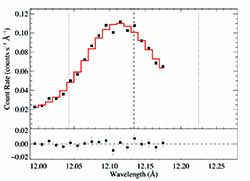
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.37e-3   +/- (:) q = 0 hinf = 2 (frozen) taustar = 3.42   +/- (2.81:4.29) Ro = 1.60   +/- (1.47:1.74) shift = 0 norm = 3.11e-4   +/- (3.02e-4:3.19e-4) chisq = 24.19 for N = 19

iso-porous: RGS2, h_inf = 5

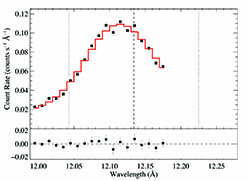
[11.98:12.18] vinf = 2250 β = 1 powerlaw continuum, n = 2 norm = 1.12e-3   +/- (:) q = 0 hinf = 5 (frozen) taustar = 12.99   +/- (8.87:19.61) Ro = 1.58   +/- (1.46:1.71) shift = 0 norm = 3.16e-4   +/- (3.08e-4:3.24e-4) chisq = 26.31 for N = 19

Summarizing

We show the 68%, 95%, and 99% limits below. The best-fit model is the filled circle.

And here is an extensive log of all these xspec fits.

 

Back to the top

---

last modified: 10 May 2012